What is Covariance?

What is covariance?

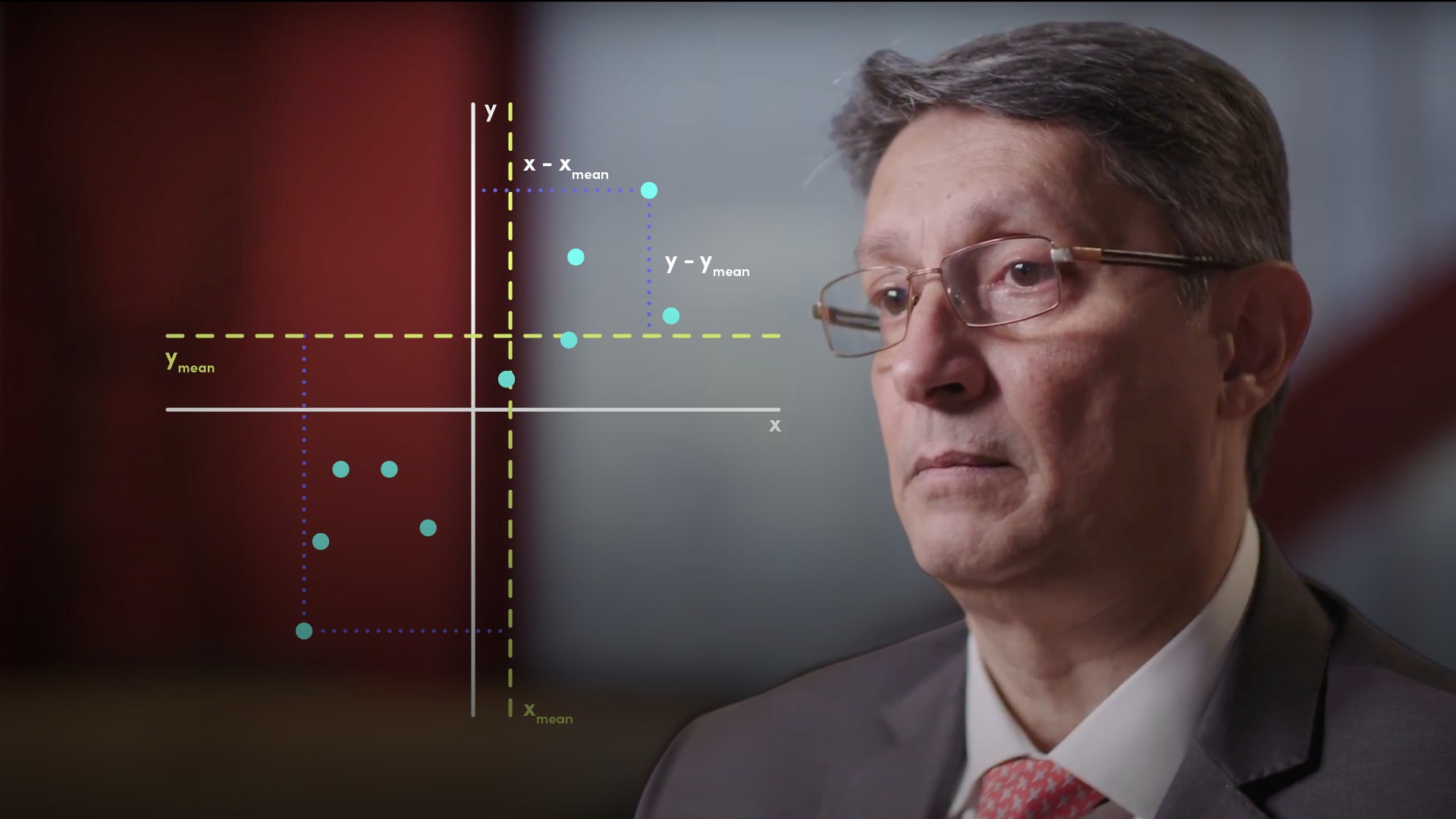
The first thing we need to do is find the average daily return for two different assets, that’s x-mean and y-mean. Next, for each data point subtract the mean from the actual return for both x and y.  Then, multiply the two and repeat for each data point and add up all those products. Finally, divide by n, if you consider the data to be the entire population, or n minus one if you consider the data set as being a sample from a larger set of data. That’s the covariance. The result will be either a positive number or a negative number and it’s the sign that’s important.

Cov(xy) = Σ (X - X_mean) (Y - Y_mean)/n

n - 1 for sample

What is the significance of covariance?

• A positive covariance means that they tend to go up and down at the same time, but not always.
• A negative covariance means that they tend to move in opposite directions, but not always.

The magnitude of the number is not important. If prices were used as inputs, one asset might have a high price, the other a low one, or both might have high prices. The resulting number will be dependent on the size of the inputs and therefore not relevant or useful.

What are the limitations of covariance?

The main drawback with covariance is that it gives us a binary result, positive or negative. It doesn’t allow us to identify the area where, in reality, we would consider the relationship to be weak or non-existent. It doesn't tell us anything about the strength of that relationship. We leave that to correlation, and covariance is a stepping stone to correlation. Nor does it tell us anything about the size of the relationship, by that we mean if gold moves by 1%, by how much would we expect the Euro-Dollar rate to move. We leave that to linear regression.